What are Significant Figures? Definition
Sig figs are all the digits that are additional to the magnitude of a number. To avert repetitive figures that are not significant, you can round the given number.
However, you have to be very careful lest you end up losing precision while rounding. Most of the time, rounding numbers is meant for simplicity only.
Sig Fig Rules
To determine which of the numbers are significant and which ones are not, you can use the sig fig calculator or the rules of the Significant figure listed below:
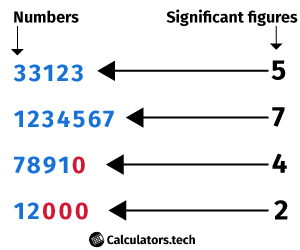
The ‘0’ to the left of the decimal value is not considered significant.
Leading zeros that occur before the first decimal number are not considered significant figures according to the rules of sig fig counter.
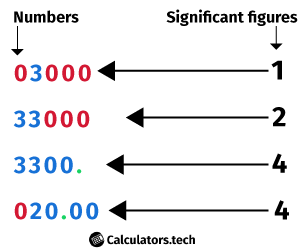
All of the trailing zeros that are place-holders, are not to be considered significant except when the decimal point is specified.
In many contexts, trailing zeros are only displayed if they are significant: for instance, if a gauging that is precise to four decimal places (0.0001) were to be given as 12.23, then it would usually be mistaken to show that just the two of decimal places of precision are available according to the rules and formula of sig figs calculator.
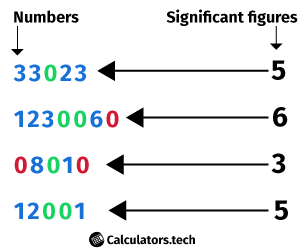
Zeros that occur between non-zero digits are significant.
let's find out how many sig figs are in 1101 with a significant figures calculator:
According to the rule of sig fig calculator, "All non-zero numbers are considered as the significant numbers" there are 3 sig figs. numbers when we combine both rules then we will get the correct answer which is 4.

All non-zero number values are significant.
It is a very simple rule that all numbers from 1-9 are considered significant digits.
for example: ( 011234567890 )
in the above example, there are 11 digits but have only 9 significant numbers.
How to Round Significant Figures?
Rounding significant figures come into play when you go for mixed calculations - addition/subtraction and multiplication/division - you need to round the value for each step of calculations to the correct number of significant figures.
For instance, to calculate: \(13.14 + 2.82 \times 2.5\),
After first step you’d obtain the following result: \(13.14 + 7.05\).
Then, you have to round the result of multiplication to 2 significant figures. Now, just add the numbers and leave two significant figures, attaining the result of \(13.14 + 7.05 = 20.19 = 20\).
Rounding Rules of Significant Figures Calculator
- When rounding off numbers to a certain value of significant figures, do so to the closest value.
Example 1:
Round to 3 significant figures: \(2.3578 \times 10^2 \)
\( \mathrm{Answer:} 2.36 \times 10^4\)
Example 2:
Round to 2 significant figures: \(1.534 \times 10^5 \)
\( \mathrm{Answer:} 1.5 \times 10^3 \)
Example 3:
Round 3663900 to 3 significant figures:
\( \mathrm{Answer:} \) 36600000
- What to do if you encounter a 5?
Sig fig calculator is an arbitrary approach to that: If the figure before 5 is odd, round up. If the figure before 5 is even, then just let it be. The argument for this is that in the path of sequences of many calculations, any errors in rounding would be averaged out due to the rules of sig figs calculator.
Significant Figures Counter Table:
Following is the table in which you can find how many significant figures are in the given number, no. of significant figures and which figures are significant. It'll help you to understand the solution of results of Ssgnificant figures calculator.
| How many Significant Figures are in? | No. of Significant Figures | Significant Figures |
| 100 | 1 | 1 |
| 100. | 3 | 1,0,0 |
| 1000 | 1 | 1 |
| 1000. | 4 | 1,0,0,0 |
| 1500 | 2 | 1,5 |
| 210 | 2 | 2,1 |
| 0.056 | 2 | 5,6 |
| 400 | 1 | 4 |
| 0.00120 | 3 | 1,2,0 |
| 0.123 | 3 | 1,2,3 |
| 207.52 | 5 | 2,0,7,5,2 |
| 5780 | 3 | 5,7,8 |
| 0.001070 | 4 | 1,0,7,0 |
| 2600.38 | 6 | 2,6,0,0,3,8 |
| 4.05 | 3 | 4,0,5 |
| 0.0560 | 3 | 5, 6, 0 |
FAQ
How to Count Significant Figures?
There are following 3 basic rules to count the number of significant figures into a number.
Digits which has no any zero are always significant.
- If two significant digits contain one or more than one zeros, then that numbers are significant.
- If the decimal portion of a number complain trailing zeros or final zero, then it is significant.
You can easily calculate significant numbers with details by using our Sig Fig Calculator.
How Many Sig Figs in 100?
There is only one sig fig number in 100 and it is 1. Because according to the rules of the sig fig counter, there is no any zero in decimals. That is why we can’t count zeros as a significant number.
How Many Sig Figs is 10.0?
All digits of the given number are significant because 10.0 has 3 sig fig digits and 1 decimal number. For easy calculation, we can count the number of sig figs in the given equation of 10.0. All 3 digits should be counted from beginning to end because there is no insignificant digit in it.
How Many Sig Figs are in 1000?
According to the significant figures calculator, all zeros in the given number are not significant because these are not decimals. So, 1 is the only sig fig number.
References
Wikipedia - Significant figures
Chemistry in the Community; Kendall-Hunt: Dubuque, IA 1988
- Engelbrecht, Nancy; et al. (1990). "Rounding Decimal Numbers to a Designated Precision" (PDF). Washington, D.C.: U.S. Department of Education.